Many are probably familiar with Alan Turing’s radical work in the field of computation and artificial intelligence. But, my favourite work of Turing’s is his single biology paper published about the morphogenics and the growth of organisms. I have been slowly reading through it over the past week, something I had intended to do for a long while. The man is a natural writer and an seamless presenter of clear ideas. For those interested in reading this work you can find it here: The Chemical Basis of Morphogenesis. Turing is gentle through the entire paper and takes care to guide the reader to sections that suits their abilities well. I will not be covering the paper because I wish to keep these journals short. Instead I want to highlight a quote which wrangled my attention as it solved a long-lasting question I had about the field of embryology. Having spoken to a few biologists, none were able to answer my question, which I thought must have been rather simple for an expert in the field. The question was as follows:

If mammals (it could be all Eukaryotes, but I chose mammals to simplify the scope) start from a single cell, of which divides into two cells, then four cells, then eight cells and so on, and each division is symmetrical, i.e. when two cells divide into four, presumably the four cells are indistinguishable from each other as the two cells would have been from each other and so to the single cell, then how do asymmetries form?

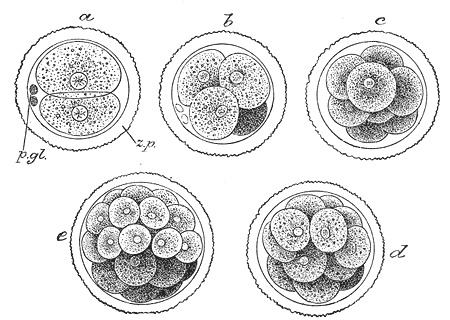
It is a question of symmetry breaking. Assuming the cell is rotationally invariant (and invariant to a range of other transformations possibly) on average, then during cellular division, on average, all cells should be relatively similar, that is there is no way to distinguish between any two cells at any time in the process. There are, of course, some initial answers one might imagine. Could the concentrations in the cell be asymmetrical, therefore the original claim about symmetrical cell division is a fallacy? Perhaps the cell’s environment is asymmetrical, with different molecular concentrations at its ‘top’ instead of its ‘bottom’. Or could gravity impose an asymmetry (I discarded this option, but I could be wrong)? None could give me a satisfing answer that closed the question to further debate.

Hence, I was extatic when Turing posed my exact question in his paper. Turing writes:

“An embryo in its spherical blastula stage has spherical symmetry, or if there are an deviations from perfect symmetry, they cannot be regarded as of any particular importance, for the deviations vary greatly from embryo to embryo within a species, though the organisms developed from them are barely distinguishable. One may take it therefore that there is perfect spherical symmetry. But a system which has spherical symmetry, and whose state is changing because of chemical reactions and diffusion, will remain spherically symmetrical for ever. (The same would hold true if the state were chaning according to the laws of electricity and magnetism, or of quantum mechanics). It certainly cannot result in an organism such as a horse, which is not spherically symmetrical.” (Turing, 1952)

..and immediately we are told there is a “fallacy” in asking such a question. An answer is coming! Turing’s answer:

” It was assumed that the deviations from the spherical symmetry in the blastula could be ignored because it makes no particular difference what form of asymmetry there is. It is, however, important that there are some deviations, for the system may reach a state of instability in which these irregularities, or certain components of them, tend to grow.”

Two paragraphs later he then describes a scenario of two cells in which they are homogenous, that is, they both have the same concentration. He prompts his answer with a description of how the concentrations of two types of morphogens (Turing’s name for the molecules that drive morphogenics) at each cell will diffuse between both cells and react according to a set of simple equations he defines. A reading of this section 4 of his paper is highly encouraged. But, Turing concludes by showing that if just a small perturbation occcurred such that the concentrations were very very very slightly off from one another, then the dynamics of the system evolve into a nonhomogenous state and equilibrium disappears.

Please read Turing’s paper if you find a spare weekend. The man is able to weave a story while performing thorough scientific analysis and the reaction-diffusion system he creates by the end of it has wonderful applications. See below.

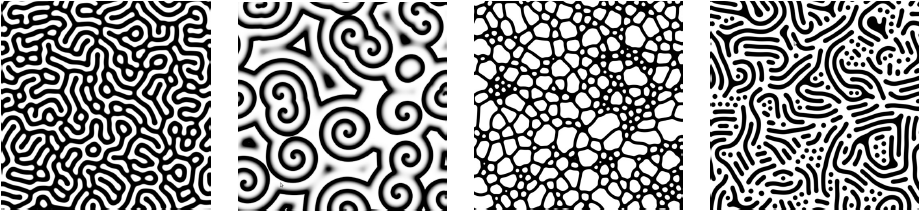
(first image courtesy of Karl Sims tutorial page on reaction-diffusion systems) (second image courtesy of this biology course)